Hillcrest Amphitheater

Here are three quick questions concerning the Hillcrest Amphitheater in the Lyle School of Engineering on the campus of Southern Methodist University. First, if you laid a plank of wood on top of the seats, and another plank on top of the steps, and a third on top of the hand rails, which would have the greatest slope? Second, what fraction of a circle does the the amphitheater take up? Third, what point in the amphitheater is equidistant from the entire first row of seats?

These questions should work well to spark geometric conversations among tour participants. Try to allow them time to come to their own answers. When the group discussions have completed, come together and see if you agree with the following conclusions:

On the first, all of the planks will have the same slope. The railing is parallel to the steps because it is always the same height above the steps (all of the support posts are the same height), and parallel lines have the same slope. Also, from the picture we can see that each step is one-third the height of each of the seats, and if you measure, you will find that each one is also one-third the depth. Therefore the slope, which is the rise (or the height) divided by the run (or depth) is the same for the steps as the seats.

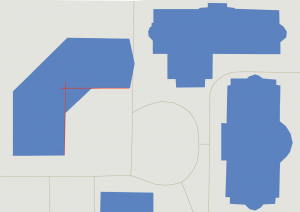
On the second, we can see (although not in the above picture) that the amphitheater is tucked between two adjacent walls of the building it is attached to. Since building walls are generally placed at right angles, we conclude that the amphitheater is a quarter circle.

On the third, how do we even know there is a point equidistant from all of the seats in the first row? Well, each row is a circular arc, and that’s exactly what a circle is, the set of points equidistant from a single point, the center of the circle. So we need to find the center of the amphitheater circle. For that, we can use the principle that the center of a circle is the intersection of any two radii. Harkening back to the previous question, notice that the two walls of the building defining the two boundaries of the amphitheater are necessarily radii of the amphitheater. So armed with the map below, we can see that the point we’re looking for is actually inside the building — not as good a place to address a crowd at Hillcrest Amphitheater about the wonders of math as we might have hoped!